Lösung 2.2:5c

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (Lösning 2.2:5c moved to Solution 2.2:5c: Robot: moved page)
Zeile 1: Zeile 1:
 +
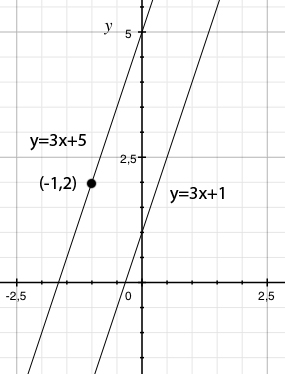
Two straight lines are parallel if they have the same gradient. From the line
 +
<math>y=3x+1</math>, we can read off that it has a gradient of
 +
<math>3</math>
 +
(the coefficient in front of
 +
<math>x</math>
 +
), and hence the equation we are looking for has an equation of the form
 +
 +
 +
<math>y=3x+m</math>
 +
 +
 +
where
 +
<math>m</math>
 +
is a constant. The condition that the line should also contain the point
 +
<math>\left( -1 \right.,\left. 2 \right)</math>
 +
means that the point should satisfy the equation of the line
 +
 +
 +
<math>2=3\left( -1 \right)+m</math>
 +
 +
 +
which gives
 +
<math>m=5</math>. Hence, the equation of the line is
 +
<math>y=3x+5</math>.
 +
 +
{{NAVCONTENT_START}}
{{NAVCONTENT_START}}
[[Image:S1_2_2_5_c.jpg]]
[[Image:S1_2_2_5_c.jpg]]
<!--<center> [[Image:2_2_5c.png]] </center>-->
<!--<center> [[Image:2_2_5c.png]] </center>-->
{{NAVCONTENT_STOP}}
{{NAVCONTENT_STOP}}

Version vom 09:25, 18. Sep. 2008

Two straight lines are parallel if they have the same gradient. From the line \displaystyle y=3x+1, we can read off that it has a gradient of \displaystyle 3 (the coefficient in front of \displaystyle x ), and hence the equation we are looking for has an equation of the form


\displaystyle y=3x+m


where \displaystyle m is a constant. The condition that the line should also contain the point \displaystyle \left( -1 \right.,\left. 2 \right) means that the point should satisfy the equation of the line


\displaystyle 2=3\left( -1 \right)+m


which gives \displaystyle m=5. Hence, the equation of the line is \displaystyle y=3x+5.


Image:S1_2_2_5_c.jpg

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_2.2:5c“