Lösung 4.1:6c

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
(Sprache und Formulierung)
Zeile 5: Zeile 5:
bringen, wo wir direkt den Mittelpunkt <math>\left( a \right.,\left. b \right)</math> und den Radius <math>r</math> ablesen können.
bringen, wo wir direkt den Mittelpunkt <math>\left( a \right.,\left. b \right)</math> und den Radius <math>r</math> ablesen können.
-
Wir bringen zuerst den Faktor <math>3</math> aus den Klammern heraus
+
Wir klammern zuerst den Faktor <math>3</math> aus:
<math>\begin{align}
<math>\begin{align}
Zeile 12: Zeile 12:
\end{align}</math>
\end{align}</math>
-
und dividieren danach beide Seiten mit 9, und erhalten so die Gleichung
+
und dividieren danach beide Seiten durch 9. So erhalten wir die Gleichung
<math>\left( x-\frac{1}{3} \right)^{2}+\left( y+\frac{7}{3} \right)^{2}=\frac{10}{9}</math>
<math>\left( x-\frac{1}{3} \right)^{2}+\left( y+\frac{7}{3} \right)^{2}=\frac{10}{9}</math>
Zeile 18: Zeile 18:
Nachdem die rechte Seite
Nachdem die rechte Seite
<math>\left( \sqrt{\frac{10}{9}} \right)^{2}</math>
<math>\left( \sqrt{\frac{10}{9}} \right)^{2}</math>
-
ist, und der term
+
ist und der Term
<math>\left( y+\frac{7}{3} \right)^{2}</math>
<math>\left( y+\frac{7}{3} \right)^{2}</math>
-
wie
+
als
-
<math></math>
+
<math>\left( y-\left( -\frac{7}{3} \right) \right)^{2}\,.</math>
-
geschrieben werden kann, erhalten wir die Gleichung
+
geschrieben werden kann, erhalten wir eine Gleichung in obiger Form.
-
 
+
-
<math>\left( y-\left( -\frac{7}{3} \right) \right)^{2}</math>
+
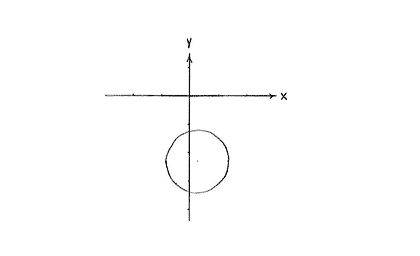
Dies ist die Gleichung eines Kreises mit dem Mittelpunkt
Dies ist die Gleichung eines Kreises mit dem Mittelpunkt
<math>\left( \frac{1}{3} \right.,\left. -\frac{7}{3} \right)</math>
<math>\left( \frac{1}{3} \right.,\left. -\frac{7}{3} \right)</math>
und dem Radius
und dem Radius
-
<math>\sqrt{\frac{10}{9}}=\frac{\sqrt{10}}{3}</math>
+
<math>\sqrt{\frac{10}{9}}=\frac{\sqrt{10}}{3}\,.</math>
{{NAVCONTENT_START}}
{{NAVCONTENT_START}}
<center> [[Image:4_1_6c-2(2).gif]] </center>
<center> [[Image:4_1_6c-2(2).gif]] </center>
{{NAVCONTENT_STOP}}
{{NAVCONTENT_STOP}}

Version vom 13:51, 16. Jun. 2009

Wir müssen die Gleichung des Kreises auf die Form

\displaystyle \left( x-a \right)^{2}+\left( y-b \right)^{2}=r^{2}

bringen, wo wir direkt den Mittelpunkt \displaystyle \left( a \right.,\left. b \right) und den Radius \displaystyle r ablesen können.

Wir klammern zuerst den Faktor \displaystyle 3 aus:

\displaystyle \begin{align} & \left( 3x-1 \right)^{2}+\left( 3y+7 \right)^{2}=3^{2}\left( x-\frac{1}{3} \right)^{2}+3^{2}\left( y+\frac{7}{3} \right)^{2} \\ & =9\left( x-\frac{1}{3} \right)^{2}+9\left( y+\frac{7}{3} \right)^{2} \\ \end{align}

und dividieren danach beide Seiten durch 9. So erhalten wir die Gleichung

\displaystyle \left( x-\frac{1}{3} \right)^{2}+\left( y+\frac{7}{3} \right)^{2}=\frac{10}{9}

Nachdem die rechte Seite \displaystyle \left( \sqrt{\frac{10}{9}} \right)^{2} ist und der Term \displaystyle \left( y+\frac{7}{3} \right)^{2} als \displaystyle \left( y-\left( -\frac{7}{3} \right) \right)^{2}\,. geschrieben werden kann, erhalten wir eine Gleichung in obiger Form.

Dies ist die Gleichung eines Kreises mit dem Mittelpunkt \displaystyle \left( \frac{1}{3} \right.,\left. -\frac{7}{3} \right) und dem Radius \displaystyle \sqrt{\frac{10}{9}}=\frac{\sqrt{10}}{3}\,.

Image:4_1_6c-2(2).gif
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.1:6c“