Lösung 4.2:1e

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K (hat „Solution 4.2:1e“ nach „Lösung 4.2:1e“ verschoben: Robot: moved page)
Zeile 1: Zeile 1:
-
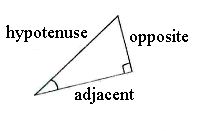
In the triangle, we seek the hypotenuse ''x'', knowing the angle 35° and that the adjacent has length 11.
+
Ersucht ist die Hypotenuse ''x'', und wir wissen den Winkel 35° und die länge der Ankathete.
[[Image:4_2_1_e.gif|center]]
[[Image:4_2_1_e.gif|center]]
-
The definition of sine gives
+
Durch die Definition von Sinus erhalten wir
{{Abgesetzte Formel||<math>\sin 35^{\circ} = \frac{11}{x}</math>}}
{{Abgesetzte Formel||<math>\sin 35^{\circ} = \frac{11}{x}</math>}}
-
and thus
+
und also
{{Abgesetzte Formel||<math>x = \frac{11}{\sin 35^{\circ}}\quad ({} \approx 19\textrm{.}2)\,\textrm{.}</math>}}
{{Abgesetzte Formel||<math>x = \frac{11}{\sin 35^{\circ}}\quad ({} \approx 19\textrm{.}2)\,\textrm{.}</math>}}

Version vom 11:53, 4. Apr. 2009

Ersucht ist die Hypotenuse x, und wir wissen den Winkel 35° und die länge der Ankathete.

Durch die Definition von Sinus erhalten wir

\displaystyle \sin 35^{\circ} = \frac{11}{x}

und also

\displaystyle x = \frac{11}{\sin 35^{\circ}}\quad ({} \approx 19\textrm{.}2)\,\textrm{.}
Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.2:1e“