Aus Online Mathematik Brückenkurs 1

Version vom 14:46, 22. Okt. 2008 (bearbeiten) Tekbot (Diskussion | Beiträge) K (hat „Solution 4.1:4c“ nach „Lösung 4.1:4c“ verschoben: Robot: moved page) ← Zum vorherigen Versionsunterschied		Version vom 17:17, 2. Apr. 2009 (bearbeiten) (rückgängig) Martin (Diskussion | Beiträge) Zum nächsten Versionsunterschied →
Zeile 1:		Zeile 1:
-	Let the point on the ''x''-axis have coordinates <math>(x,0)</math>, where ''x'' is an unknown number. Then, using the distance formula, the distance from the point	+	Die ''x''-Achse besteht aus allen Punkten <math>(x,0)</math>, wo ''x'' unbekannt ist. Mit der Formel für den Abstand zwischen zwei Punkten, erhalten wir den Abstand zwischen <math>(x,0)</math> und <math>(3,3)</math> und zwischen <math>(5,1)</math> und <math>(x,0)</math>,
-	<math>(x,0)</math> to <math>(3,3)</math> and <math>(5,1)</math> is given by	+
-	{{Abgesetzte Formel||<math>\sqrt{(x-3)^2+(0-3)^2}\qquad\text{and}\qquad \sqrt{(x-5)^2+(0-1)^2}\,\textrm{,}</math>}}	+	{{Abgesetzte Formel||<math>\sqrt{(x-3)^2+(0-3)^2}\qquad\text{und}\qquad \sqrt{(x-5)^2+(0-1)^2}\,\textrm{,}</math>}}
-	respectively. Because these two distances should be the same, we get the equation	+	Nachdem die beiden Abstände gleich sein sollen, erhalten wir
	{{Abgesetzte Formel||<math>\sqrt{(x-3)^2+9} = \sqrt{(x-5)^2+1}</math>}}		{{Abgesetzte Formel||<math>\sqrt{(x-3)^2+9} = \sqrt{(x-5)^2+1}</math>}}
-	or, if we take the square, so as to get rid of the square root sign,	+	Wir quadrieren diese Gleichung und erhalten
	{{Abgesetzte Formel||<math>(x-3)^2 + 9 = (x-5)^2+1\,\textrm{.}</math>}}		{{Abgesetzte Formel||<math>(x-3)^2 + 9 = (x-5)^2+1\,\textrm{.}</math>}}
-	Now, expand the squares and collect together all the terms onto one side,	+	Wir erweitern alle Terme, und sammeln danach alle Terme auf einer Seite,
	{{Abgesetzte Formel||<math>\begin{align}		{{Abgesetzte Formel||<math>\begin{align}
Zeile 19:		Zeile 18:
	\end{align}</math>}}		\end{align}</math>}}
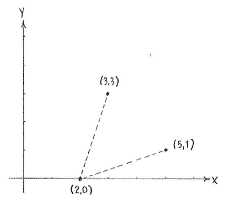
-	This gives that <math>x=2</math>, i.e. the point on the ''x''-axis is <math>(2,0)\,</math>.	+	Dies ergibt <math>x=2</math>, und also ist der Punkt auf der ''x''-Achse <math>(2,0)\,</math>.
	[[Image:4_1_4_c-1(2).gif|center]]		[[Image:4_1_4_c-1(2).gif|center]]
-		+	Zum Schluss kontrollieren wir ob die beiden Abstände auch wirklich gleich sind. Der Abstand zwischen <math>(2,0)</math> und <math>(3,3)</math>
-	As a final step, we check that we have calculated correctly and that the distances really are the same. The distance between <math>(2,0)</math> and <math>(3,3)</math>	+	ist
-	is	+
	{{Abgesetzte Formel||<math>\sqrt{(3-2)^2 + (3-0)^2} = \sqrt{1^2+3^2} = \sqrt{1+9} = \sqrt{10}</math>}}		{{Abgesetzte Formel||<math>\sqrt{(3-2)^2 + (3-0)^2} = \sqrt{1^2+3^2} = \sqrt{1+9} = \sqrt{10}</math>}}
-	and the distance between <math>(2,0)</math> and <math>(5,1)</math> is	+	und der Abstand zwischen <math>(2,0)</math> und <math>(5,1)</math> ist
	{{Abgesetzte Formel||<math>\sqrt{(5-2)^2 + (1-0)^2} = \sqrt{3^2 + 1^2} = \sqrt{9+1} = \sqrt{10}\,\textrm{.}</math>}}		{{Abgesetzte Formel||<math>\sqrt{(5-2)^2 + (1-0)^2} = \sqrt{3^2 + 1^2} = \sqrt{9+1} = \sqrt{10}\,\textrm{.}</math>}}
-		+	Hinweis: Obwohl wir unsere Gleichung quadriert haben, besteht kein Risiko Scheingleichungen zu erhalten, nachdem die Quadraten in der Wurzel immer positiv sind.
-	Note: Although we squared our root equation, it is not in fact necessary to test the solution for that reason, because the expressions under the root signs are sums of squares which are never negative and therefore cannot give rise to so-called spurious roots.	+

---

Version vom 17:17, 2. Apr. 2009

Die x-Achse besteht aus allen Punkten \displaystyle (x,0), wo x unbekannt ist. Mit der Formel für den Abstand zwischen zwei Punkten, erhalten wir den Abstand zwischen \displaystyle (x,0) und \displaystyle (3,3) und zwischen \displaystyle (5,1) und \displaystyle (x,0),

\displaystyle \sqrt{(x-3)^2+(0-3)^2}\qquad\text{und}\qquad \sqrt{(x-5)^2+(0-1)^2}\,\textrm{,}

Nachdem die beiden Abstände gleich sein sollen, erhalten wir

\displaystyle \sqrt{(x-3)^2+9} = \sqrt{(x-5)^2+1}

Wir quadrieren diese Gleichung und erhalten

\displaystyle (x-3)^2 + 9 = (x-5)^2+1\,\textrm{.}

Wir erweitern alle Terme, und sammeln danach alle Terme auf einer Seite,

\displaystyle \begin{align} & x^2-6x+9+9 = x^2-10x+25+1\\[5pt] &\quad\Leftrightarrow\quad 4x-8=0\,\textrm{.} \end{align}

Dies ergibt \displaystyle x=2, und also ist der Punkt auf der x-Achse \displaystyle (2,0)\,.

Zum Schluss kontrollieren wir ob die beiden Abstände auch wirklich gleich sind. Der Abstand zwischen \displaystyle (2,0) und \displaystyle (3,3) ist

\displaystyle \sqrt{(3-2)^2 + (3-0)^2} = \sqrt{1^2+3^2} = \sqrt{1+9} = \sqrt{10}

und der Abstand zwischen \displaystyle (2,0) und \displaystyle (5,1) ist

\displaystyle \sqrt{(5-2)^2 + (1-0)^2} = \sqrt{3^2 + 1^2} = \sqrt{9+1} = \sqrt{10}\,\textrm{.}

Hinweis: Obwohl wir unsere Gleichung quadriert haben, besteht kein Risiko Scheingleichungen zu erhalten, nachdem die Quadraten in der Wurzel immer positiv sind.