Lösung 4.4:5c
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
K (hat „Solution 4.4:5c“ nach „Lösung 4.4:5c“ verschoben: Robot: moved page) |
Version vom 15:16, 22. Okt. 2008
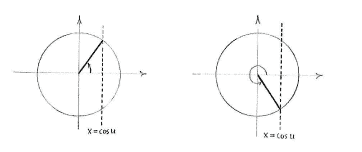
For a fixed value of u, an equality of the form
| \displaystyle \cos u=\cos v |
is satisfied by two angles v in the unit circle,
| \displaystyle v=u\qquad\text{and}\qquad v=-u\,\textrm{.} |
This means that all angles v which satisfy the equality are
| \displaystyle v=u+2n\pi\qquad\text{and}\qquad v=-u+2n\pi\,, |
where n is an arbitrary integer.
Therefore, the equation
| \displaystyle \cos 5x=\cos (x+\pi/5) |
has the solutions
| \displaystyle \left\{\begin{align} 5x&=x+\frac{\pi}{5}+2n\pi\quad\text{or}\\[5pt] 5x &= -x-\frac{\pi}{5}+2n\pi\,\textrm{.}\end{align}\right. |
If we collect x onto one side, we end up with
| \displaystyle \left\{\begin{align}
x &= \frac{\pi}{20} + \frac{n\pi}{2}\,,\\[5pt] x &= -\frac{\pi }{30}+\frac{n\pi}{3}\,, \end{align}\right. |
where n is an arbitrary integer.