Lösung 2.3:10b
Aus Online Mathematik Brückenkurs 1
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
|||
| Zeile 26: | Zeile 26: | ||
Note: If you feel unsure about whether the parabola really does lie under the line, i.e. that it just happens to look as though it does, we can investigate if the ''y''-values on the line <math>y_{\scriptstyle\text{line}} = x/2+3/2</math> is always larger than the corresponding ''y''-value on the parabola <math>y_{\scriptstyle\text{parabola}} = 1-x^{2}</math> by studying the difference between them | Note: If you feel unsure about whether the parabola really does lie under the line, i.e. that it just happens to look as though it does, we can investigate if the ''y''-values on the line <math>y_{\scriptstyle\text{line}} = x/2+3/2</math> is always larger than the corresponding ''y''-value on the parabola <math>y_{\scriptstyle\text{parabola}} = 1-x^{2}</math> by studying the difference between them | ||
| - | {{ | + | {{Abgesetzte Formel|| |
<math>y_{\scriptstyle\text{line}} - y_{\scriptstyle\text{parabola}} = \frac{x}{2} + \frac{3}{2} - (1-x^{2})\,\textrm{.}</math>}} | <math>y_{\scriptstyle\text{line}} - y_{\scriptstyle\text{parabola}} = \frac{x}{2} + \frac{3}{2} - (1-x^{2})\,\textrm{.}</math>}} | ||
If this difference is positive regardless of how ''x'' is chosen, then we know that the line's ''y''-value is always greater than the parabola's ''y''-value. After a little simplification and completing the square, we have | If this difference is positive regardless of how ''x'' is chosen, then we know that the line's ''y''-value is always greater than the parabola's ''y''-value. After a little simplification and completing the square, we have | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
y_{\scriptstyle\text{line}} - y_{\scriptstyle\text{parabola}} | y_{\scriptstyle\text{line}} - y_{\scriptstyle\text{parabola}} | ||
&= \frac{x}{2} + \frac{3}{2} - (1-x^{2})\\[5pt] | &= \frac{x}{2} + \frac{3}{2} - (1-x^{2})\\[5pt] | ||
Version vom 08:30, 22. Okt. 2008
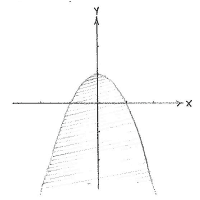
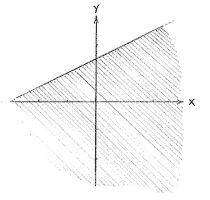
The inequality \displaystyle y\le 1-x^{2} defines the area under and on the curve \displaystyle y=1-x^{2}, which is a parabola with a maximum at (0,1). We can rewrite the other inequality \displaystyle x\ge 2y-3 as \displaystyle y\le x/2+3/2 and it defines the area under and on the straight line \displaystyle y=x/2+3/2.
| The region y ≤ 1 - x² | The region x ≥ 2y - 3 |
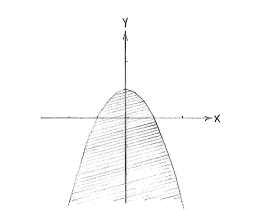
Of the figures above, it seems that the region associated with the parabola lies completely under the line \displaystyle y=x/2+3/2 and this means that the area under the parabola satisfies both inequalities.
| The region y ≤ 1 - x² and x ≥ 2y - 3 |
Note: If you feel unsure about whether the parabola really does lie under the line, i.e. that it just happens to look as though it does, we can investigate if the y-values on the line \displaystyle y_{\scriptstyle\text{line}} = x/2+3/2 is always larger than the corresponding y-value on the parabola \displaystyle y_{\scriptstyle\text{parabola}} = 1-x^{2} by studying the difference between them
|
\displaystyle y_{\scriptstyle\text{line}} - y_{\scriptstyle\text{parabola}} = \frac{x}{2} + \frac{3}{2} - (1-x^{2})\,\textrm{.} |
If this difference is positive regardless of how x is chosen, then we know that the line's y-value is always greater than the parabola's y-value. After a little simplification and completing the square, we have
| \displaystyle \begin{align}
y_{\scriptstyle\text{line}} - y_{\scriptstyle\text{parabola}} &= \frac{x}{2} + \frac{3}{2} - (1-x^{2})\\[5pt] &= x^{2}+\frac{1}{2}x+\frac{1}{2}\\[5pt] &= \Bigl(x+\frac{1}{4}\Bigr)^{2} - \Bigl(\frac{1}{4}\Bigr)^{2} + \frac{1}{2}\\[5pt] &= \Bigl(x+\frac{1}{4}\Bigr)^{2} + \frac{7}{16} \end{align} |
and this expression is always positive because \displaystyle \tfrac{7}{16} is a positive number and \displaystyle \bigl(x+\tfrac{1}{4}\bigr)^{2} is a quadratic which is never negative. In other words, the parabola is completely under the line.