Lösung 2.2:5b
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
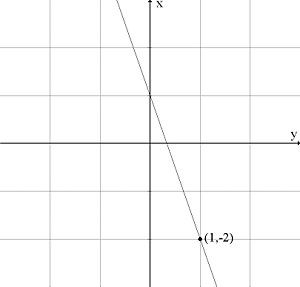
Because the straight line is to have a slope of <math>-3</math>, its equation can be written as | Because the straight line is to have a slope of <math>-3</math>, its equation can be written as | ||
| - | {{ | + | {{Abgesetzte Formel||<math>y=-3x+m\,,</math>}} |
where ''m'' is a constant. If the line is also to pass through the point (''x'',''y'') = (1,-2), the point must satisfy the equation of the line, | where ''m'' is a constant. If the line is also to pass through the point (''x'',''y'') = (1,-2), the point must satisfy the equation of the line, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>-2=-3\cdot 1+m\,,</math>}} |
which gives that <math>m=1</math>. | which gives that <math>m=1</math>. | ||
Version vom 08:28, 22. Okt. 2008
Because the straight line is to have a slope of \displaystyle -3, its equation can be written as
| \displaystyle y=-3x+m\,, |
where m is a constant. If the line is also to pass through the point (x,y) = (1,-2), the point must satisfy the equation of the line,
| \displaystyle -2=-3\cdot 1+m\,, |
which gives that \displaystyle m=1.
The answer is thus that the equation of the line is \displaystyle y=-3x+1.