Lösung 2.2:5a
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
K (Robot: Automated text replacement (-{{Displayed math +{{Abgesetzte Formel)) |
||
| Zeile 1: | Zeile 1: | ||
Let's write down the equation for a straight line as | Let's write down the equation for a straight line as | ||
| - | {{ | + | {{Abgesetzte Formel||<math>y=kx+m\,,</math>}} |
where ''k'' and ''m'' are constants which we shall determine. | where ''k'' and ''m'' are constants which we shall determine. | ||
| Zeile 7: | Zeile 7: | ||
Since the points (2,3) and (3,0) should lie on the line, they must also satisfy the equation of the line, | Since the points (2,3) and (3,0) should lie on the line, they must also satisfy the equation of the line, | ||
| - | {{ | + | {{Abgesetzte Formel||<math>3=k\cdot 2+m\qquad\text{and}\qquad 0=k\cdot 3+m\,\textrm{.}</math>}} |
If we take the difference between the equations, ''m'' disappears and we can work out the slope ''k'', | If we take the difference between the equations, ''m'' disappears and we can work out the slope ''k'', | ||
| - | {{ | + | {{Abgesetzte Formel||<math>\begin{align} |
3-0 &= k\cdot 2+m-(k\cdot 3+m)\,,\\[5pt] | 3-0 &= k\cdot 2+m-(k\cdot 3+m)\,,\\[5pt] | ||
3 &= -k\,\textrm{.} | 3 &= -k\,\textrm{.} | ||
| Zeile 18: | Zeile 18: | ||
Substituting this into the equation <math>0=k\centerdot 3+m</math> then gives us a value for ''m'', | Substituting this into the equation <math>0=k\centerdot 3+m</math> then gives us a value for ''m'', | ||
| - | {{ | + | {{Abgesetzte Formel||<math>m=-3k=-3\cdot (-3)=9\,\textrm{.}</math>}} |
The equation of the line is thus <math>y=-3x+9</math>. | The equation of the line is thus <math>y=-3x+9</math>. | ||
Version vom 08:28, 22. Okt. 2008
Let's write down the equation for a straight line as
| \displaystyle y=kx+m\,, |
where k and m are constants which we shall determine.
Since the points (2,3) and (3,0) should lie on the line, they must also satisfy the equation of the line,
| \displaystyle 3=k\cdot 2+m\qquad\text{and}\qquad 0=k\cdot 3+m\,\textrm{.} |
If we take the difference between the equations, m disappears and we can work out the slope k,
| \displaystyle \begin{align}
3-0 &= k\cdot 2+m-(k\cdot 3+m)\,,\\[5pt] 3 &= -k\,\textrm{.} \end{align} |
Substituting this into the equation \displaystyle 0=k\centerdot 3+m then gives us a value for m,
| \displaystyle m=-3k=-3\cdot (-3)=9\,\textrm{.} |
The equation of the line is thus \displaystyle y=-3x+9.
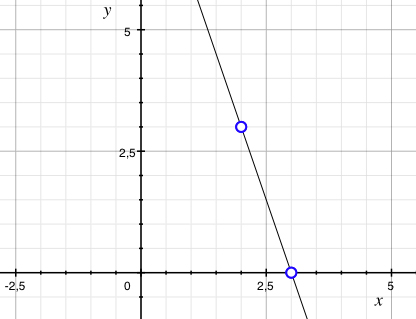
Note. To be completely certain that we have calculated correctly, we check that the points (2,3) and (3,0) satisfy the equation of the line:
- (x,y) = (2,3): \displaystyle \text{LHS} = 3\ and \displaystyle \ \text{RHS} = -3\cdot 2+9 = 3\,.
- (x,y) = (3,0): \displaystyle \text{LHS} = 0\ and \displaystyle \ \text{LHS} = -3\cdot 3+9 = 0\,.
