Lösung 4.2:7
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
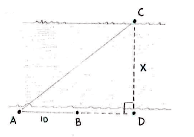
| - | If extend the line | + | If we extend the line AB to a point D opposite C, we will get the right-angled triangle shown below, where the distance ''x'' between C and D is the desired distance. |
| - | + | ||
| - | to a point | + | |
| - | + | ||
| - | opposite | + | |
| - | + | ||
| - | + | ||
| - | between | + | |
| - | + | ||
| - | and | + | |
| - | + | ||
| - | is the desired distance. | + | |
| - | + | ||
[[Image:4_2_7_1.gif|center]] | [[Image:4_2_7_1.gif|center]] | ||
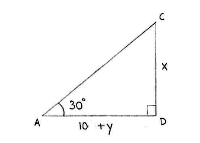
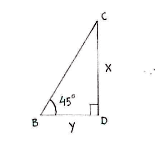
| - | The information in the exercise can be summarized by considering the two triangles | + | The information in the exercise can be summarized by considering the two triangles ACD and BCD, and setting up relations for the tangents that the angles 30° and 45° gives rise to, |
| - | + | ||
| - | and | + | |
| - | + | ||
| - | + | ||
| - | and | + | |
| - | + | ||
| - | gives rise to, | + | |
| + | {| align="center" | ||
| + | |align="center"|[[Image:4_2_7_2-1.gif]] | ||
| + | |width="20px"| | ||
| + | |align="center"|[[Image:4_2_7_2-2.gif]] | ||
| + | |- | ||
| + | |align="center" valign="top"|<math>\begin{align} x &= (10+y)\tan 30^{\circ}\\[5pt] &= (10+y)\frac{1}{\sqrt{3}}\end{align}</math> | ||
| + | || | ||
| + | |align="center" valign="top"|<math>\begin{align} x &= y\cdot\tan 45^{\circ}\\[5pt] &= y\cdot 1\end{align}</math> | ||
| + | |} | ||
| - | + | where ''y'' is the distance between B and D. | |
| - | <math> | + | The second relation above gives that <math>y=x</math> and substituting this into the first relation gives |
| + | {{Displayed math||<math>x = (10+x)\frac{1}{\sqrt{3}}\,\textrm{.}</math>}} | ||
| + | Multiplying both sides by <math>\sqrt{3}</math> gives | ||
| - | + | {{Displayed math||<math>\sqrt{3}x=10+x</math>}} | |
| - | <math> | + | |
| - | + | ||
| - | + | moving all the ''x''-terms to the left-hand side gives | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | moving all the x-terms to the left-hand side gives | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| + | {{Displayed math||<math>(\sqrt{3}-1)x = 10\,\textrm{.}</math>}} | ||
The answer is | The answer is | ||
| - | + | {{Displayed math||<math>x = \frac{10}{\sqrt{3}-1}\ \text{m}\approx 13\textrm{.}6\ \text{m.}</math>}} | |
| - | <math>x=\frac{ | + | |
Version vom 11:54, 9. Okt. 2008
If we extend the line AB to a point D opposite C, we will get the right-angled triangle shown below, where the distance x between C and D is the desired distance.
The information in the exercise can be summarized by considering the two triangles ACD and BCD, and setting up relations for the tangents that the angles 30° and 45° gives rise to,
| 
| |
| \displaystyle \begin{align} x &= (10+y)\tan 30^{\circ}\\[5pt] &= (10+y)\frac{1}{\sqrt{3}}\end{align} | \displaystyle \begin{align} x &= y\cdot\tan 45^{\circ}\\[5pt] &= y\cdot 1\end{align} |
where y is the distance between B and D.
The second relation above gives that \displaystyle y=x and substituting this into the first relation gives
Multiplying both sides by \displaystyle \sqrt{3} gives
moving all the x-terms to the left-hand side gives
The answer is