Lösung 4.2:5a

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
Zeile 1: Zeile 1:
-
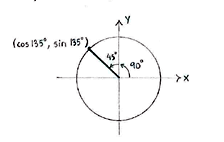
Because
+
Because <math>135^{\circ} = 90^{\circ} + 45^{\circ}</math>, <math>135^{\circ}</math> is an angle in the second quadrant which makes an angle of <math>45^{\circ}</math> with the positive ''y''-axis.
-
<math>\text{135}^{\circ }\text{ }=\text{ 9}0^{\circ }\text{ }+\text{45}^{\circ }</math>,
+
-
<math>\text{135}^{\circ }\text{ }</math>
+
-
is an angle in the second quadrant which makes an angle of
+
-
<math>\text{45}^{\circ }</math>
+
-
with the positive
+
-
<math>y</math>
+
-
-axis.
+
-
 
+
[[Image:4_2_5_a1.gif|center]]
[[Image:4_2_5_a1.gif|center]]
-
We can determine the point on the unit circle which corresponds to
+
We can determine the point on the unit circle which corresponds to <math>135^{\circ}</math> by introducing an auxiliary triangle and calculating its edges using trigonometry.
-
<math>\text{135}^{\circ }\text{ }</math>
+
-
by introducing an auxiliary triangle and calculating its edges using trigonometry.
+
-
 
+
-
 
+
-
[[Image:4_2_5_a2.gif|center]]
+
-
 
+
-
opposite<math>=1\centerdot \sin \centerdot 45^{\circ }=\frac{1}{\sqrt{2}}</math>
+
-
 
+
-
adjacent
+
-
<math>=1\centerdot \cos \centerdot 45^{\circ }=\frac{1}{\sqrt{2}}</math>
+
 +
{| width="100%"
 +
|width="50%" align="center"|[[Image:4_2_5_a2.gif|center]]
 +
|width="50%" align="left"|<math>\begin{align}\text{opposite} &= 1\cdot\sin 45^{\circ} = \dfrac{1}{\sqrt{2}}\\[5pt] \text{adjacent} &= 1\cdot\cos 45^{\circ} = \frac{1}{\sqrt{2}}\end{align}</math>
 +
|}
-
The coordinates of the point are
+
The coordinates of the point are <math>( -1/\!\sqrt{2}, 1/\!\sqrt{2})</math> and this shows that <math>\cos 135^{\circ} = -1/\!\sqrt{2}\,</math>.
-
<math>\left( -\frac{1}{\sqrt{2}} \right.,\left. \frac{1}{\sqrt{2}} \right)</math>
+
-
and this shows that
+
-
<math>\text{cos135}^{\circ }=-\frac{1}{\sqrt{2}}</math>.
+

Version vom 11:01, 9. Okt. 2008

Because \displaystyle 135^{\circ} = 90^{\circ} + 45^{\circ}, \displaystyle 135^{\circ} is an angle in the second quadrant which makes an angle of \displaystyle 45^{\circ} with the positive y-axis.

We can determine the point on the unit circle which corresponds to \displaystyle 135^{\circ} by introducing an auxiliary triangle and calculating its edges using trigonometry.

\displaystyle \begin{align}\text{opposite} &= 1\cdot\sin 45^{\circ} = \dfrac{1}{\sqrt{2}}\\[5pt] \text{adjacent} &= 1\cdot\cos 45^{\circ} = \frac{1}{\sqrt{2}}\end{align}

The coordinates of the point are \displaystyle ( -1/\!\sqrt{2}, 1/\!\sqrt{2}) and this shows that \displaystyle \cos 135^{\circ} = -1/\!\sqrt{2}\,.

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/L%C3%B6sung_4.2:5a“