Lösung 4.1:9
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
| - | + | 10 seconds corresponds to 1/6 minutes, so that during that time period, the second hand sweeps over 1/6 of a turn, i.e. the sector of a circle with angle | |
| - | seconds corresponds to | + | |
| - | + | ||
| - | minutes, so that during that time period, the second hand sweeps over | + | |
| - | + | ||
| - | of a turn, i.e. the sector of a circle with angle | + | |
| + | {{Displayed math||<math>\alpha = \frac{1}{6}\cdot 2\pi\ \text{radians} = \frac{\pi}{3}\ \text{radians.}</math>}} | ||
| - | <math>\alpha =\frac{1}{6}\centerdot 2\pi </math> | ||
| - | radians | ||
| - | <math>=\frac{\pi }{3}</math> | ||
| - | radians | ||
| - | |||
| - | |||
| - | {{NAVCONTENT_START}} | ||
<center> [[Image:4_1_9_.gif]] </center> | <center> [[Image:4_1_9_.gif]] </center> | ||
| - | |||
| - | |||
| - | {{NAVCONTENT_STOP}} | ||
The area of the sector is | The area of the sector is | ||
| - | + | {{Displayed math||<math>\text{Area} = \frac{1}{2}\alpha r^{2} = \frac{1}{2}\cdot \frac{\pi}{3}\cdot (8\ \text{cm})^2 = \frac{32\pi}{3}\ \text{cm}^{2} \approx 33\textrm{.}5\ \text{cm}^{2}\,\textrm{.}</math>}} | |
| - | <math>=\frac{1}{2}\alpha r^{2}=\frac{1}{2}\ | + | |
Version vom 11:50, 8. Okt. 2008
10 seconds corresponds to 1/6 minutes, so that during that time period, the second hand sweeps over 1/6 of a turn, i.e. the sector of a circle with angle
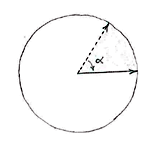
The area of the sector is
