Lösung 2.3:10a
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K |
|||
| Zeile 1: | Zeile 1: | ||
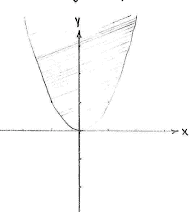
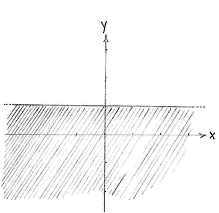
| - | Individually, the inequalities | + | Individually, the inequalities <math>y\ge x^{2}</math> and <math>y\le 1</math> define the region above the parabola <math>y=x^{2}</math> and under the line <math>y=1</math>, respectively. |
| - | <math>y\ge x^ | + | |
| - | and | + | {| align="center" |
| - | <math>y\le | + | |align="center"|[[Image:2_3_10_a-1.gif|center]] |
| - | define the region above the parabola | + | |width="10px"| |
| - | <math>y=x^ | + | |align="center"|[[Image:2_3_10_a-2.gif|center]] |
| - | and under the line | + | |- |
| - | <math>y= | + | |align="center"|<small>The region ''y'' ≥ ''x''²</small> |
| + | || | ||
| + | |align="center"|<small>The region ''y'' ≤ 1</small> | ||
| + | |} | ||
| + | |||
| + | Those points which satisfy both inequalities lie in the region above the parabola, but below the line <math>y=1\,</math>. | ||
| - | [[Image:2_3_10_a.gif|center]] | ||
| - | Those points which satisfy both inequalities lie in the region above the parabola, but below the line | ||
| - | <math>y=\text{1}</math> | ||
[[Image:2_3_10_a2.gif|center]] | [[Image:2_3_10_a2.gif|center]] | ||
Version vom 14:25, 29. Sep. 2008
Individually, the inequalities \displaystyle y\ge x^{2} and \displaystyle y\le 1 define the region above the parabola \displaystyle y=x^{2} and under the line \displaystyle y=1, respectively.
| The region y ≥ x² | The region y ≤ 1 |
Those points which satisfy both inequalities lie in the region above the parabola, but below the line \displaystyle y=1\,.