Lösung 4.2:1a
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (Lösning 4.2:1a moved to Solution 4.2:1a: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
| - | {{NAVCONTENT_START}} | ||
| - | <center> [[Image:4_2_1a.gif]] </center> | ||
| - | {{NAVCONTENT_STOP}} | ||
[[Image:4_2_1_a.gif|center]] | [[Image:4_2_1_a.gif|center]] | ||
| + | |||
| + | |||
| + | |||
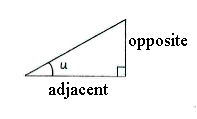
| + | The definition of the tangent states that | ||
| + | |||
| + | |||
| + | |||
| + | <math>\tan u=\frac{\text{opposite}}{\text{adjacent}}</math> | ||
| + | |||
| + | |||
| + | In our case, this means that | ||
| + | |||
| + | |||
| + | <math>\tan 27^{\circ }=\frac{x}{13}</math> | ||
| + | |||
| + | |||
| + | which gives | ||
| + | <math>x=\text{13}\centerdot \text{tan 27}^{\circ }</math>. | ||
| + | |||
| + | NOTE: Using a calculator, we can work out what | ||
| + | <math>x\text{ }</math> | ||
| + | should be: | ||
| + | |||
| + | |||
| + | <math>x=\text{13}\centerdot \text{tan 27}^{\circ }\approx 6.62</math> | ||
Version vom 10:27, 28. Sep. 2008
The definition of the tangent states that
\displaystyle \tan u=\frac{\text{opposite}}{\text{adjacent}}
In our case, this means that
\displaystyle \tan 27^{\circ }=\frac{x}{13}
which gives
\displaystyle x=\text{13}\centerdot \text{tan 27}^{\circ }.
NOTE: Using a calculator, we can work out what \displaystyle x\text{ } should be:
\displaystyle x=\text{13}\centerdot \text{tan 27}^{\circ }\approx 6.62