Lösung 2.3:8b
Aus Online Mathematik Brückenkurs 1
(Unterschied zwischen Versionen)
K (Lösning 2.3:8b moved to Solution 2.3:8b: Robot: moved page) |
|||
| Zeile 1: | Zeile 1: | ||
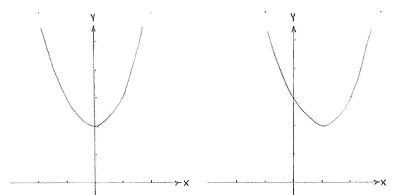
| - | { | + | As a starting point, we can take the curve |
| - | < | + | <math>y=x^{2}+2</math> |
| - | {{ | + | which is a parabola with a minimum at |
| + | <math>\left( 0 \right.,\left. 2 \right)</math> | ||
| + | and is sketched further down. Compared with that curve, | ||
| + | <math>y=\left( x-1 \right)^{2}+2</math> | ||
| + | is the same curve in which we must consistently choose | ||
| + | <math>x</math> | ||
| + | to be one unit greater in order to get the same | ||
| + | <math>y</math> | ||
| + | -value. The curve | ||
| + | <math>y=\left( x-1 \right)^{2}+2</math> | ||
| + | is thus shifted one unit to the right compared with | ||
| + | <math>y=x^{2}+2</math>. | ||
| + | |||
| + | |||
[[Image:2_3_8_b.gif|center]] | [[Image:2_3_8_b.gif|center]] | ||
Version vom 11:30, 21. Sep. 2008
As a starting point, we can take the curve \displaystyle y=x^{2}+2 which is a parabola with a minimum at \displaystyle \left( 0 \right.,\left. 2 \right) and is sketched further down. Compared with that curve, \displaystyle y=\left( x-1 \right)^{2}+2 is the same curve in which we must consistently choose \displaystyle x to be one unit greater in order to get the same \displaystyle y -value. The curve \displaystyle y=\left( x-1 \right)^{2}+2 is thus shifted one unit to the right compared with \displaystyle y=x^{2}+2.