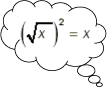Processing Math: 69%
To print higher-resolution math symbols, click the
Hi-Res Fonts for Printing button on the jsMath control panel.
No jsMath TeX fonts found -- using image fonts instead.
These may be slow and might not print well.
Use the jsMath control panel to get additional information.
jsMath Control PanelHide this Message

jsMath

3.1 Wurzeln

Aus Online Mathematik Brückenkurs 1

(Unterschied zwischen Versionen)
Wechseln zu: Navigation, Suche
K
K
Zeile 20: Zeile 20:
*That the square root of a negative number has not been defined.
*That the square root of a negative number has not been defined.
*That the square root of a number denotes the positive root.
*That the square root of a number denotes the positive root.
-
*How to manipulate roots in the simplification of expressions containing roo
+
*How to manipulate roots in the simplification of expressions containing roots.
-
*To recognise when the methods of manipulating roots are valid.
+
*To recognise when the methods of manipulating roots are valid. (Non-negative radicands).
*How to simplify expressions containing quadratic roots in the denominator.
*How to simplify expressions containing quadratic roots in the denominator.
*When the n:th root of a negative number is defined (n odd).
*When the n:th root of a negative number is defined (n odd).
Zeile 31: Zeile 31:
The well-known symbol <math>\sqrt{a}</math>, the square root of <math>a</math>, is used to describe the number that when multiplied by itself gives <math>a</math>. However, one has to be a little more precise in defining this symbol.
The well-known symbol <math>\sqrt{a}</math>, the square root of <math>a</math>, is used to describe the number that when multiplied by itself gives <math>a</math>. However, one has to be a little more precise in defining this symbol.
-
The equation <math>x^2 = 4</math> has two solutions <math>x = 2</math> and <math>x = -2</math>, since both <math>2\cdot 2 = 4</math> and <math>(-2)\cdot(-2) = 4</math>. It would then be logical to suppose that <math>\sqrt{4}</math> can be either <math>-2</math> or <math>2</math>, dvs. <math>\sqrt{4}= \pm 2</math>, but <math>\sqrt{4}</math> only denotes the positive number <math>2</math>.
+
The equation <math>x^2 = 4</math> has two solutions <math>x = 2</math> and <math>x = -2</math>, since both <math>2\cdot 2 = 4</math> and <math>(-2)\cdot(-2) = 4</math>. It would then be logical to suppose that <math>\sqrt{4}</math> can be either <math>-2</math> or <math>2</math>, i.e. <math>\sqrt{4}= \pm 2</math>, but <math>\sqrt{4}</math> only denotes the positive number <math>2</math>.
Zeile 123: Zeile 123:
Note that, unlike quadratics roots, cube roots are also defined for negative numbers.
Note that, unlike quadratics roots, cube roots are also defined for negative numbers.
-
For any positive integers <math>n</math> one can define the the n:th root of a number<math>a</math> as
+
For any positive integers <math>n</math> one can define the the n:th root of a number <math>a</math> as
* if <math>n</math> is even and <math>a\ge0</math> then <math>\sqrt[\scriptstyle n]{a}</math> is the non-negative number that when multiplied by itself <math>n</math> times gives <math>a</math>,
* if <math>n</math> is even and <math>a\ge0</math> then <math>\sqrt[\scriptstyle n]{a}</math> is the non-negative number that when multiplied by itself <math>n</math> times gives <math>a</math>,
Zeile 319: Zeile 319:
-
'''Länktips'''
+
'''Useful web sites'''
[http://mathforum.org/dr.math/faq/faq.sqrt.by.hand.html How to find the root of a number, without the help of calculators?]
[http://mathforum.org/dr.math/faq/faq.sqrt.by.hand.html How to find the root of a number, without the help of calculators?]
</div>
</div>

Version vom 08:47, 7. Aug. 2008

 

Vorlage:Vald flik Vorlage:Ej vald flik

 

Content:

  • Quadratic roots and n:th roots
  • Manipulating roots

Learning outcomes:

After this section, you will have learned:

  • How to calculate the square root of some simple integers.
  • That the square root of a negative number has not been defined.
  • That the square root of a number denotes the positive root.
  • How to manipulate roots in the simplification of expressions containing roots.
  • To recognise when the methods of manipulating roots are valid. (Non-negative radicands).
  • How to simplify expressions containing quadratic roots in the denominator.
  • When the n:th root of a negative number is defined (n odd).

Quadratic roots

The well-known symbol a , the square root of a, is used to describe the number that when multiplied by itself gives a. However, one has to be a little more precise in defining this symbol.

The equation x2=4 has two solutions x=2 and x=−2, since both 22=4 and (−2)(−2)=4. It would then be logical to suppose that 4  can be either −2 or 2, i.e. 4=2 , but 4  only denotes the positive number 2.


Square root a  "means the non-negative number that multiplied by itself gives a i.e. the non-negative solution to the equation x2=a.

Square root of a can also be written as a12 .

It is therefore wrong to state that 4=2  but correct to state that the equation x2=4 has the solution x=2.

Example 1

  1. 0=0  because 02=00=0 and 0 is not negative.
  2. 100=10  since 102=1010=100 and 10 is a positive number.
  3. 025=05  since 052=0505=025 and 05 is positive.
  4. 214142  since 14142141422 and 14142 is positive.
  5. The equation x2=2 has the solutions x=21414  and x=−2−1414 .
  6. −4  is not defined, since there is no real number x that satisfies x2=−4.
  7. (−7)2=7  because (−7)2=(−7)(−7)=49=77=7 .

When taking square roots, it is useful to know some methods of calculation. As a=a12  we can use the laws of exponents as "laws of roots" For example, we have Vorlage:Fristående formel In this way we obtain the following rules for quadratic roots, which applies to all real numbers ab0:

Vorlage:Fristående formel

( We must however, in the above division, assume as always that b is not 0.)

Example 2

  1. 6481=6481=89=72 
  2. 925=925=53 
  3. 182=182=36=6 
  4. 375=375=25=5 
  5. 12=43=43=23 

Note that the above calculations assume that a and b0. If a and b are negative (< 0) then a  and b  are not defined as real numbers. It is tempting to write , for example,

Vorlage:Fristående formel

but something here cannot be right. The explanation is that −1  is not a real number, which means the laws of roots discussed above may not be used.


Higher order roots

The cube root of a number a is defined as the number that multiplied by itself three times gives a, and is denoted as 3a .

Example 3

  1. 38=2  as 222=8.
  2. 30027=03  since 030303=0027.
  3. 3−8=−2  because (−2)(−2)(−2)=−8.

Note that, unlike quadratics roots, cube roots are also defined for negative numbers.

For any positive integers n one can define the the n:th root of a number a as

  • if n is even and a0 then na  is the non-negative number that when multiplied by itself n times gives a,
  • if n is odd, na  is the number that when multiplied by itself n times gives a.

The root na  can also be written as a1n .

Example 4

  1. 4625=5  since 5555=625.
  2. \displaystyle \sqrt[\scriptstyle 5]{-243} = -3\quad because \displaystyle (-3) \cdot (-3) \cdot (-3) \cdot (-3) \cdot (-3) = -243.
  3. \displaystyle \sqrt[\scriptstyle 6]{-17}\quad is not defined as \displaystyle 6 is even and \displaystyle -17 is a negative number.

For \displaystyle n:th roots the same rules apply as for quadratic roots if \displaystyle a, \, b \ge 0. Note that if \displaystyle n is odd these methods apply even for negative \displaystyle a and \displaystyle b, that is, for all real numbers \displaystyle a and \displaystyle b.

Vorlage:Fristående formel


Simplification of expressions containing roots

Often, one can significantly simplify expressions containing roots by using the usual methods for roots . As is also the case when using the laws of exponents, it is desirable to reduce expressions into as "small" roots as possible. For example, it is a good idea to do the following

Vorlage:Fristående formel

because it helps simplification as we see here

Vorlage:Fristående formel

By rewriting expressions containing roots in terms of "small" roots one can also sum roots of "the same kind", e.g.

Vorlage:Fristående formel

Example 5

  1. \displaystyle \frac{\sqrt{8}}{\sqrt{18}} = \frac{\sqrt{2 \cdot 4}}{\sqrt{2 \cdot 9}} = \frac{\sqrt{2 \cdot 2 \cdot 2}}{\sqrt{2 \cdot 3 \cdot 3}} = \frac{\sqrt{2 \cdot 2^2}}{\sqrt{2 \cdot 3^2}} = \frac{2\sqrt{2}}{3\sqrt{2}} = \frac{2}{3}
  2. \displaystyle \frac{\sqrt{72}}{6} = \frac{\sqrt{8 \cdot 9}}{ 2 \cdot 3} = \frac{\sqrt{2 \cdot 2 \cdot 2 \cdot 3 \cdot 3}}{ 2 \cdot 3} = \frac{\sqrt{2^2 \cdot 3^2 \cdot 2}}{ 2 \cdot 3} = \frac{2 \cdot 3\sqrt{2}}{2 \cdot 3} = \sqrt{2}
  3. \displaystyle \sqrt{45} + \sqrt{20} = \sqrt{9\cdot5} + \sqrt{4\cdot5} = \sqrt{3^2\cdot5} + \sqrt{2^2\cdot5} = 3\sqrt{5} + 2\sqrt{5}\vphantom{\bigl(}
    \displaystyle \phantom{\sqrt{45} + \sqrt{20}\vphantom{\bigl(}}{} = (3+2)\sqrt{5} = 5\sqrt{5}
  4. \displaystyle \sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(} = \sqrt{5 \cdot 10} + 2\sqrt{3} -\sqrt{2 \cdot 16} + \sqrt{3 \cdot 9}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = \sqrt{5 \cdot 2 \cdot 5} + 2\sqrt{3} -\sqrt{2 \cdot 4 \cdot 4} + \sqrt{3 \cdot 3 \cdot 3}\vphantom{a^{b^c}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = \sqrt{5^2 \cdot 2 } + 2\sqrt{3} -\sqrt{2^2 \cdot 2^2 \cdot 2} + \sqrt{3 \cdot 3^2}\vphantom{a^{\textstyle b^{\textstyle c}}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = 5\sqrt{2} +2\sqrt{3} - 2 \cdot 2\sqrt{2} + 3\sqrt{3}\vphantom{a^{\textstyle b^{\textstyle c}}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = (5-4)\sqrt{2} + (2+3)\sqrt{3}\vphantom{a^{\textstyle b^{\textstyle c}}}
    \displaystyle \phantom{\sqrt{50} + 2\sqrt{3} -\sqrt{32} + \sqrt{27}\vphantom{\Bigl(}}{} = \sqrt{2} + 5\sqrt{3}\vphantom{a^{\textstyle b^{\textstyle c}}}
  5. \displaystyle \frac{ 2\cdot\sqrt[\scriptstyle3]{3} }{ \sqrt[\scriptstyle3]{12} } = \frac{ 2\cdot\sqrt[\scriptstyle3]{3} }{ \sqrt[\scriptstyle3]{3 \cdot 4} } = \frac{ 2\cdot\sqrt[\scriptstyle3]{3} }{ \sqrt[\scriptstyle3]{3} \cdot \sqrt[\scriptstyle3]{4} } = \frac{ 2 }{ \sqrt[\scriptstyle3]{4} } = \frac{ 2 }{ \sqrt[\scriptstyle3]{2 \cdot 2} } = \frac{ 2 }{ \sqrt[\scriptstyle3]{2} \cdot \sqrt[\scriptstyle3]{2} } \cdot \displaystyle \frac{\sqrt[\scriptstyle3]{2}}{ \sqrt[\scriptstyle3]{2}} = \frac{ 2\cdot\sqrt[\scriptstyle3]{2} }{ 2 } = \sqrt[\scriptstyle3]{2}
  6. \displaystyle (\sqrt{3} + \sqrt{2}\,)(\sqrt{3} - \sqrt{2}\,) = (\sqrt{3}\,)^2-(\sqrt{2}\,)^2 = 3-2 = 1 where we have used the difference of two squares \displaystyle (a+b)(a-b) = a^2 - b^2 with \displaystyle a=\sqrt{3} and \displaystyle b=\sqrt{2}.


Rational root expressions

When roots appear in a rational expression one often want to avoid roots in the denominator (because it is difficult with hand calculations to divide by irrational numbers). By multiplying the numerator and denominator by \displaystyle \sqrt{2} for example, one obtains

Vorlage:Fristående formel

which usually is preferable.

In other cases, you can take advantage of the difference of two squares method, \displaystyle (a+b)(a-b) = a^2 – b^2. One multiplies the numerator and denominator by the denominators “conjugate” and the root sign is eliminated from the denominator by squaring, as in the following,

Vorlage:Fristående formel

Example 6

  1. \displaystyle \frac{10\sqrt{3}}{\sqrt{5}} = \frac{10\sqrt{3}\cdot\sqrt{5}}{\sqrt{5}\cdot\sqrt{5}} = \frac{10\sqrt{15}}{5} = 2\sqrt{15}
  2. \displaystyle \frac{1+\sqrt{3}}{\sqrt{2}} = \frac{(1+\sqrt{3})\cdot\sqrt{2}}{\sqrt{2}\cdot\sqrt{2}} = \frac{\sqrt{2}+\sqrt{6}}{2}
  3. \displaystyle \frac{3}{\sqrt{2}-2} = \frac{3(\sqrt{2}+2)}{(\sqrt{2}-2)(\sqrt{2}+2)} = \frac{3\sqrt{2}+6}{(\sqrt{2}\,)^2-2^2} = \frac{3\sqrt{2}+6}{2-4} = -\frac{3\sqrt{2}+6}{2}
  4. \displaystyle \frac{\sqrt{2}}{\sqrt{6}+\sqrt{3}} = \frac{\sqrt{2}\,(\sqrt{6}-\sqrt{3}\,)}{(\sqrt{6}+\sqrt{3}\,) (\sqrt{6}-\sqrt{3}\,)} = \frac{\sqrt{2}\,\sqrt{6}-\sqrt{2}\,\sqrt{3}}{(\sqrt{6}\,)^2 -(\sqrt{3}\,)^2}\vphantom{\Biggl(}
    \displaystyle \phantom{\frac{\sqrt{2}}{\sqrt{6}+\sqrt{3}}\vphantom{\Biggl(}}{} = \frac{\sqrt{2}\,\sqrt{2\cdot 3}-\sqrt{2}\,\sqrt{3}}{6-3} = \frac{2\sqrt{3}-\sqrt{2}\,\sqrt{3}}{3} = \frac{(2-\sqrt{2}\,)\sqrt{3}}{3} \vphantom{\displaystyle\frac{a^{\textstyle b^{\textstyle c}}}{b}}


Exercises


Study advice

Basic and final tests

After you have read the text and worked through the exercises, you should do the basic and final tests to pass this section. You can find the link to the tests in your student lounge.

Keep in mind that:

Square root of a number is always non-negative (that is positive or zero)!

Rules for roots are actually special case of laws of exponents .

For example: \displaystyle \sqrt{x}=x^{1/2}.


Reviews

For those of you who want to deepen your studies or need more detailed explanations consider the following references

Learn more about square roots in the English Wikipedia

How do we know that the root of 2 is not a fraction?


Useful web sites

How to find the root of a number, without the help of calculators?

Von „http://wiki.sommarmatte.se/wikis/2009/bridgecourse1-TU-Berlin/index.php/3.1_Wurzeln“