From Förberedande kurs i matematik 1

Revision as of 09:38, 9 September 2008 (edit) Tekbot (Talk | contribs) m (Lösning 4.1:7c moved to Solution 4.1:7c: Robot: moved page) ← Previous diff		Current revision (11:31, 8 October 2008) (edit) (undo) Tek (Talk | contribs) m
(One intermediate revision not shown.)
Line 1:		Line 1:
-	{{NAVCONTENT_START}}	+	By completing the square, we can rewrite the ''x''- and ''y''-terms as quadratic expressions,
-	<center> [[Image:4_1_7_c.gif]] </center>	+	{{Displayed math||<math>\begin{align}
		+	x^2 - 2x &= (x-1)^2 - 1^2\,,\\[5pt]
		+	y^2 + 6y &= (y+3)^2 - 3^2\,,
		+	\end{align}</math>}}
-	<center> [[Image:4_1_7c.gif]] </center>	+	and the whole equation then has standard form,
-	{{NAVCONTENT_STOP}}	+
		+	{{Displayed math||<math>\begin{align}
		+	(x-1)^2 - 1 + (y+3)^2 - 9 &= -3\,,\\[5pt]
		+	(x-1)^2 + (y+3)^2 &= 7\,\textrm{.}
		+	\end{align}</math>}}
		+
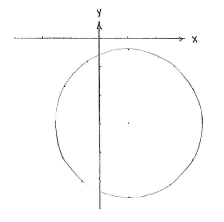
		+	From this, we see that the circle has its centre at (1,-3) and radius <math>\sqrt{7}\,</math>.
		+
		+
		+	<center> [[Image:4_1_7_c.gif]] </center>

---

Current revision

By completing the square, we can rewrite the x- and y-terms as quadratic expressions,

\displaystyle \begin{align} x^2 - 2x &= (x-1)^2 - 1^2\,,\\[5pt] y^2 + 6y &= (y+3)^2 - 3^2\,, \end{align}

and the whole equation then has standard form,

\displaystyle \begin{align} (x-1)^2 - 1 + (y+3)^2 - 9 &= -3\,,\\[5pt] (x-1)^2 + (y+3)^2 &= 7\,\textrm{.} \end{align}

From this, we see that the circle has its centre at (1,-3) and radius \displaystyle \sqrt{7}\,.