From Förberedande kurs i matematik 1

Revision as of 15:09, 15 August 2008 (edit) Madde (Talk | contribs) ← Previous diff		Current revision (08:37, 9 October 2008) (edit) (undo) Tek (Talk | contribs) m
(3 intermediate revisions not shown.)
Line 1:		Line 1:
-	{{NAVCONTENT_START}}	+	We start by subtracting <math>2\pi</math> from <math>11\pi/3</math>, so that we get an angle between <math>0</math> and <math>2\pi </math>. This doesn't change the cosine value
-	<center> [[Bild:4_2_4b-1(2).gif]] </center>	+
-	{{NAVCONTENT_STOP}}	+	{{Displayed math||<math>\cos\frac{11\pi}{3} = \cos\Bigl(\frac{11\pi}{3}-2\pi\Bigr) = \cos\frac{5\pi}{3}\,\textrm{.}</math>}}
-	{{NAVCONTENT_START}}	+
-	<center> [[Bild:4_2_4b-2(2).gif]] </center>	+	Then, by rewriting <math>5\pi/3</math> as a sum of <math>\pi</math>- and <math>\pi/2</math>-terms,
-	{{NAVCONTENT_STOP}}	+
-	[[Bild:4_2_4b1.gif]]	+	{{Displayed math||<math>\frac{5\pi}{3} = \frac{3\pi +\dfrac{3}{2}\pi +\dfrac{1}{2}\pi}{3} = \pi + \frac{\pi}{2} + \frac{\pi}{6}</math>}}
-	[[Bild:4_2_4_b2.gif]]	+
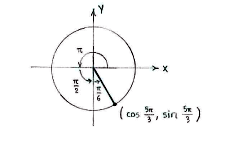
		+	we see that <math>5\pi/3</math> is an angle in the fourth quadrant which makes an angle <math>\pi/6</math> with the negative ''y''-axis.
		+
		+	[[Image:4_2_4b1.gif||center]]
		+
		+	With the help of an auxiliary triangle and a little trigonometry, we can determine the coordinates for the point on a unit circle which corresponds to the angle
		+	<math>5\pi/3\,</math>.
		+
		+	{| width="100%"
		+	|width="50%" align="center"|[[Image:4_2_4_b2.gif]]
		+	|width="50%" align="left"|<math>\begin{align}\text{opposite} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{adjacent} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}</math>
		+	|}
		+
		+	The point has coordinates <math>(1/2,-\sqrt{3}/2)</math> and
		+
		+	{{Displayed math||<math>\cos \frac{11\pi}{3} = \cos\frac{5\pi}{3} = \frac{1}{2}\,\textrm{.}</math>}}

---

Current revision

We start by subtracting \displaystyle 2\pi from \displaystyle 11\pi/3, so that we get an angle between \displaystyle 0 and \displaystyle 2\pi . This doesn't change the cosine value

\displaystyle \cos\frac{11\pi}{3} = \cos\Bigl(\frac{11\pi}{3}-2\pi\Bigr) = \cos\frac{5\pi}{3}\,\textrm{.}

Then, by rewriting \displaystyle 5\pi/3 as a sum of \displaystyle \pi- and \displaystyle \pi/2-terms,

\displaystyle \frac{5\pi}{3} = \frac{3\pi +\dfrac{3}{2}\pi +\dfrac{1}{2}\pi}{3} = \pi + \frac{\pi}{2} + \frac{\pi}{6}

we see that \displaystyle 5\pi/3 is an angle in the fourth quadrant which makes an angle \displaystyle \pi/6 with the negative y-axis.

With the help of an auxiliary triangle and a little trigonometry, we can determine the coordinates for the point on a unit circle which corresponds to the angle \displaystyle 5\pi/3\,.

\displaystyle \begin{align}\text{opposite} &= 1\cdot\sin\frac{\pi}{6} = \frac{1}{2}\\[5pt] \text{adjacent} &= 1\cdot\cos\frac{\pi}{6} = \frac{\sqrt{3}}{2}\end{align}

The point has coordinates \displaystyle (1/2,-\sqrt{3}/2) and

\displaystyle \cos \frac{11\pi}{3} = \cos\frac{5\pi}{3} = \frac{1}{2}\,\textrm{.}