Solution 2.3:10b

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
m (Lösning 2.3:10b moved to Solution 2.3:10b: Robot: moved page)
Line 1: Line 1:
-
{{NAVCONTENT_START}}
+
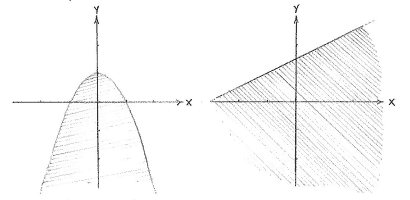
The inequality
-
<center> [[Image:2_3_10b-1(3).gif]] </center>
+
<math>y\le \text{1}-x^{\text{2}}</math>
-
{{NAVCONTENT_STOP}}
+
defines the area under and on the curve
-
{{NAVCONTENT_START}}
+
<math>y=\text{1}-x^{\text{2}}</math>, which is a parabola with a maximum at
-
<center> [[Image:2_3_10b-2(3).gif]] </center>
+
<math>\left( 0 \right.,\left. 1 \right)</math>. We can rewrite the other inequality
-
{{NAVCONTENT_STOP}}
+
<math>x\ge \text{ 2}y-\text{3}</math>
-
{{NAVCONTENT_START}}
+
as
-
<center> [[Image:2_3_10b-3(3).gif]] </center>
+
<math>y\le \text{ }{x}/{2}\;+{3}/{2}\;</math>
-
{{NAVCONTENT_STOP}}
+
and it defines the area under and on the straight line
 +
<math>y=\text{ }{x}/{2}\;+{3}/{2}\;</math>.
 +
 
 +
 
[[Image:2_3_10_b1.gif|center]]
[[Image:2_3_10_b1.gif|center]]
 +
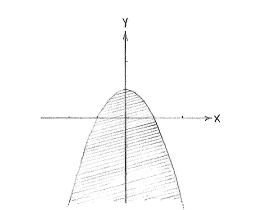
Of the figures above, it seems that the region associated with the parabola lies completely under the line
 +
<math>y=\text{ }{x}/{2}\;+{3}/{2}\;</math>
 +
and this means that the area under the parabola satisfies both inequalities.
 +
[[Image:2_3_10_b2.gif|center]]
[[Image:2_3_10_b2.gif|center]]
 +
 +
NOTE: If you feel unsure about whether the parabola really does lie under the line, i.e. that it just happens to look as though it does, we can investigate if the
 +
<math>y</math>
 +
-values on the line
 +
<math>y_{\text{line}}=\text{ }{x}/{2}\;+{3}/{2}\;</math>
 +
is always larger than the corresponding
 +
<math>y</math>
 +
-value on the parabola
 +
<math>y_{\text{parabola}}=\text{1}-x^{\text{2}}</math>
 +
by studying the difference between them:
 +
 +
 +
<math>y_{\text{line}}-y_{\text{parabola}}=\text{ }\frac{x}{2}+\frac{3}{2}-\left( \text{1}-x^{\text{2}} \right)</math>
 +
 +
 +
If this difference is positive regardless of how
 +
<math>x</math>
 +
is chosen, then we know that the line's
 +
<math>y</math>
 +
-value is always greater than the parabola's
 +
<math>y</math>
 +
-value. After a little simplification and completing the square, we have
 +
 +
 +
<math>\begin{align}
 +
& y_{\text{line}}-y_{\text{parabola}}=\text{ }\frac{x}{2}+\frac{3}{2}-\left( \text{1}-x^{\text{2}} \right)=x^{2}+\frac{1}{2}x+\frac{1}{2} \\
 +
& =\left( x+\frac{1}{4} \right)^{2}-\left( \frac{1}{4} \right)^{2}+\frac{1}{2}=\left( x+\frac{1}{4} \right)^{2}+\frac{7}{16} \\
 +
\end{align}</math>
 +
 +
 +
and this expression is always positive because
 +
<math>\frac{7}{16}</math>
 +
is a positive number and
 +
<math>\left( x+\frac{1}{4} \right)^{2}</math>
 +
is a quadratic which is never negative. In other words, the parabola is completely under the line.

Revision as of 12:39, 21 September 2008

The inequality \displaystyle y\le \text{1}-x^{\text{2}} defines the area under and on the curve \displaystyle y=\text{1}-x^{\text{2}}, which is a parabola with a maximum at \displaystyle \left( 0 \right.,\left. 1 \right). We can rewrite the other inequality \displaystyle x\ge \text{ 2}y-\text{3} as \displaystyle y\le \text{ }{x}/{2}\;+{3}/{2}\; and it defines the area under and on the straight line \displaystyle y=\text{ }{x}/{2}\;+{3}/{2}\;.


Of the figures above, it seems that the region associated with the parabola lies completely under the line \displaystyle y=\text{ }{x}/{2}\;+{3}/{2}\; and this means that the area under the parabola satisfies both inequalities.

NOTE: If you feel unsure about whether the parabola really does lie under the line, i.e. that it just happens to look as though it does, we can investigate if the \displaystyle y -values on the line \displaystyle y_{\text{line}}=\text{ }{x}/{2}\;+{3}/{2}\; is always larger than the corresponding \displaystyle y -value on the parabola \displaystyle y_{\text{parabola}}=\text{1}-x^{\text{2}} by studying the difference between them:


\displaystyle y_{\text{line}}-y_{\text{parabola}}=\text{ }\frac{x}{2}+\frac{3}{2}-\left( \text{1}-x^{\text{2}} \right)


If this difference is positive regardless of how \displaystyle x is chosen, then we know that the line's \displaystyle y -value is always greater than the parabola's \displaystyle y -value. After a little simplification and completing the square, we have


\displaystyle \begin{align} & y_{\text{line}}-y_{\text{parabola}}=\text{ }\frac{x}{2}+\frac{3}{2}-\left( \text{1}-x^{\text{2}} \right)=x^{2}+\frac{1}{2}x+\frac{1}{2} \\ & =\left( x+\frac{1}{4} \right)^{2}-\left( \frac{1}{4} \right)^{2}+\frac{1}{2}=\left( x+\frac{1}{4} \right)^{2}+\frac{7}{16} \\ \end{align}


and this expression is always positive because \displaystyle \frac{7}{16} is a positive number and \displaystyle \left( x+\frac{1}{4} \right)^{2} is a quadratic which is never negative. In other words, the parabola is completely under the line.

Retrieved from "http://wiki.sommarmatte.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/Solution_2.3:10b"