Solution 2.3:9b

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
m (Lösning 2.3:9b moved to Solution 2.3:9b: Robot: moved page)
Line 1: Line 1:
-
{{NAVCONTENT_START}}
+
The points of intersection are those points on the curve which also lie on the
-
<center> [[Image:2_3_9b-1(2).gif]] </center>
+
<math>~x</math>
-
{{NAVCONTENT_STOP}}
+
-axis, i.e. they are those points which satisfy both the equation of the curve
 +
<math>y=x^{\text{2}}-\text{5}x+\text{6}</math>
 +
and the equation of the
 +
<math>~x</math>
 +
-axis
 +
<math>y=0</math>,
 +
 
 +
 
 +
<math>\left\{ \begin{matrix}
 +
y=x^{\text{2}}-\text{5}x+\text{6} \\
 +
y=0\quad \quad \quad \quad \\
 +
\end{matrix} \right.</math>
 +
 
 +
 
 +
This system of equations gives directly that
 +
<math>y=0</math>
 +
and that
 +
<math>~x</math>
 +
must satisfy the second-order equation
 +
<math>x^{\text{2}}-\text{5}x+\text{6}=0</math>
 +
. By completing the square, we obtain that the left-hand side is
 +
 
 +
 
 +
<math>\begin{align}
 +
& x^{\text{2}}-\text{5}x+\text{6}=\left( x-\frac{5}{2} \right)^{2}-\left( \frac{5}{2} \right)^{2}+6 \\
 +
& =\left( x-\frac{5}{2} \right)^{2}-\frac{25}{4}+\frac{24}{4}=\left( x-\frac{5}{2} \right)^{2}-\frac{1}{4} \\
 +
\end{align}</math>
 +
 
 +
 
 +
and this gives that the equation has solutions
 +
 
 +
<math>x=\frac{5}{2}\pm \frac{1}{2}</math>, i.e.
 +
<math>x=\frac{5}{2}-\frac{1}{2}=\frac{4}{2}=2</math>
 +
and
 +
<math>x=\frac{5}{2}+\frac{1}{2}=\frac{6}{2}=3</math>.
 +
 
 +
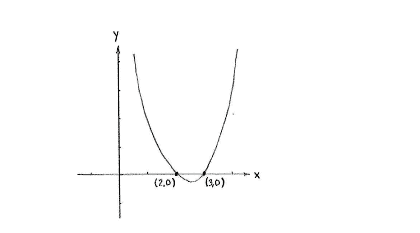
The intersection points are therefore
 +
<math>\left( 2 \right.,\left. 0 \right)</math>
 +
and
 +
<math>\left( 3 \right.,\left. 0 \right)</math>.
 +
 
 +
 
 +
 
{{NAVCONTENT_START}}
{{NAVCONTENT_START}}
<center> [[Image:2_3_9b-2(2).gif]] </center>
<center> [[Image:2_3_9b-2(2).gif]] </center>
{{NAVCONTENT_STOP}}
{{NAVCONTENT_STOP}}

Revision as of 11:57, 21 September 2008

The points of intersection are those points on the curve which also lie on the \displaystyle ~x -axis, i.e. they are those points which satisfy both the equation of the curve \displaystyle y=x^{\text{2}}-\text{5}x+\text{6} and the equation of the \displaystyle ~x -axis \displaystyle y=0,


\displaystyle \left\{ \begin{matrix} y=x^{\text{2}}-\text{5}x+\text{6} \\ y=0\quad \quad \quad \quad \\ \end{matrix} \right.


This system of equations gives directly that \displaystyle y=0 and that \displaystyle ~x must satisfy the second-order equation \displaystyle x^{\text{2}}-\text{5}x+\text{6}=0 . By completing the square, we obtain that the left-hand side is


\displaystyle \begin{align} & x^{\text{2}}-\text{5}x+\text{6}=\left( x-\frac{5}{2} \right)^{2}-\left( \frac{5}{2} \right)^{2}+6 \\ & =\left( x-\frac{5}{2} \right)^{2}-\frac{25}{4}+\frac{24}{4}=\left( x-\frac{5}{2} \right)^{2}-\frac{1}{4} \\ \end{align}


and this gives that the equation has solutions

\displaystyle x=\frac{5}{2}\pm \frac{1}{2}, i.e. \displaystyle x=\frac{5}{2}-\frac{1}{2}=\frac{4}{2}=2 and \displaystyle x=\frac{5}{2}+\frac{1}{2}=\frac{6}{2}=3.

The intersection points are therefore \displaystyle \left( 2 \right.,\left. 0 \right) and \displaystyle \left( 3 \right.,\left. 0 \right).


Image:2_3_9b-2(2).gif
Retrieved from "http://wiki.sommarmatte.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/Solution_2.3:9b"