Solution 4.4:7c

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
Current revision (07:59, 14 October 2008) (edit) (undo)
m
 
(3 intermediate revisions not shown.)
Line 1: Line 1:
-
{{NAVCONTENT_START}}
+
If we want to solve the equation <math>\cos 3x = \sin 4x</math>, we need an additional result which tells us for which values of ''u'' and ''v'' the equality
-
<center> [[Bild:4_4_7c-1(3).gif]] </center>
+
<math>\cos u = \sin v</math> holds, but to get that we have to start with the equality <math>\cos u=\cos v</math>.
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Bild:4_4_7c-2(3).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
-
{{NAVCONTENT_START}}
+
-
<center> [[Bild:4_4_7c-3(3).gif]] </center>
+
-
{{NAVCONTENT_STOP}}
+
-
[[Bild:4_4_7_c1.gif|center]]
+
So, we start by looking at the equality
-
[[Bild:4_4_7_c2.gif|center]]
+
{{Displayed math||<math>\cos u=\cos v\,\textrm{.}</math>}}
 +
 
 +
We know that for fixed ''u'' there are two angles <math>v=u</math> and <math>v=-u</math> in the unit circle which have the cosine value <math>\cos u</math>, i.e. their ''x''-coordinate is equal to <math>\cos u\,</math>.
 +
 
 +
[[Image:4_4_7_c1.gif|center]]
 +
 
 +
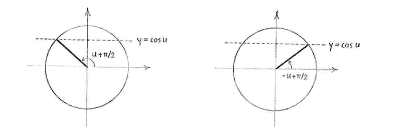
Imagine now that the whole unit circle is rotated anti-clockwise an angle <math>\pi/2</math>. The line <math>x=\cos u</math> will become the line <math>y=\cos u</math> and the angles ''u'' and -''u'' are rotated to <math>u+\pi/2</math> and <math>-u+\pi/2</math>, respectively.
 +
 
 +
[[Image:4_4_7_c2.gif|center]]
 +
 
 +
The angles <math>u+\pi/2</math> and <math>-u+\pi/2</math> therefore have their ''y''-coordinate, and hence sine value, equal to <math>\cos u</math>. In other words, the equality
 +
 
 +
{{Displayed math||<math>\cos u = \sin v</math>}}
 +
 
 +
holds for fixed ''u'' in the unit circle when <math>v = \pm u + \pi/2</math>, and more generally when
 +
 
 +
{{Displayed math||<math>v = \pm u + \frac{\pi}{2} + 2n\pi\qquad</math>(''n'' is an arbitrary integer).}}
 +
 
 +
For our equation <math>\cos 3x = \sin 4x</math>, this result means that ''x'' must satisfy
 +
 
 +
{{Displayed math||<math>4x = \pm 3x + \frac{\pi}{2} + 2n\pi\,\textrm{.}</math>}}
 +
 
 +
This means that the solutions to the equation are
 +
 
 +
{{Displayed math||<math>\left\{\begin{align}
 +
x &= \frac{\pi}{2} + 2n\pi\,,\\[5pt]
 +
x &= \frac{\pi}{14} + \frac{2}{7}\pi n\,,
 +
\end{align}\right.</math>}}
 +
 
 +
where ''n'' is an arbitrary integer.

Current revision

If we want to solve the equation \displaystyle \cos 3x = \sin 4x, we need an additional result which tells us for which values of u and v the equality \displaystyle \cos u = \sin v holds, but to get that we have to start with the equality \displaystyle \cos u=\cos v.

So, we start by looking at the equality

\displaystyle \cos u=\cos v\,\textrm{.}

We know that for fixed u there are two angles \displaystyle v=u and \displaystyle v=-u in the unit circle which have the cosine value \displaystyle \cos u, i.e. their x-coordinate is equal to \displaystyle \cos u\,.

Imagine now that the whole unit circle is rotated anti-clockwise an angle \displaystyle \pi/2. The line \displaystyle x=\cos u will become the line \displaystyle y=\cos u and the angles u and -u are rotated to \displaystyle u+\pi/2 and \displaystyle -u+\pi/2, respectively.

The angles \displaystyle u+\pi/2 and \displaystyle -u+\pi/2 therefore have their y-coordinate, and hence sine value, equal to \displaystyle \cos u. In other words, the equality

\displaystyle \cos u = \sin v

holds for fixed u in the unit circle when \displaystyle v = \pm u + \pi/2, and more generally when

\displaystyle v = \pm u + \frac{\pi}{2} + 2n\pi\qquad(n is an arbitrary integer).

For our equation \displaystyle \cos 3x = \sin 4x, this result means that x must satisfy

\displaystyle 4x = \pm 3x + \frac{\pi}{2} + 2n\pi\,\textrm{.}

This means that the solutions to the equation are

\displaystyle \left\{\begin{align}

x &= \frac{\pi}{2} + 2n\pi\,,\\[5pt] x &= \frac{\pi}{14} + \frac{2}{7}\pi n\,, \end{align}\right.

where n is an arbitrary integer.

Retrieved from "http://wiki.sommarmatte.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/Solution_4.4:7c"