Solution 4.4:2a

From Förberedande kurs i matematik 1

(Difference between revisions)
Jump to: navigation, search
m (Lösning 4.4:2a moved to Solution 4.4:2a: Robot: moved page)
Line 1: Line 1:
-
{{NAVCONTENT_START}}
+
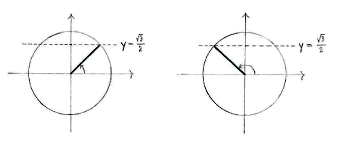
We draw a unit circle and mark on those angles on the circle which have a
-
<center> [[Image:4_4_2a-1(2).gif]] </center>
+
<math>y</math>
-
{{NAVCONTENT_STOP}}
+
-coordinate of
-
{{NAVCONTENT_START}}
+
<math>{\sqrt{3}}/{2}\;</math>, in order to see which solutions lie between
-
<center> [[Image:4_4_2a-2(2).gif]] </center>
+
<math>0</math>
-
{{NAVCONTENT_STOP}}
+
and
 +
<math>2\pi </math>.
 +
 
[[Image:4_4_2_a.gif|center]]
[[Image:4_4_2_a.gif|center]]
 +
 +
In the first quadrant, we recognize
 +
<math>x={\pi }/{3}\;</math>
 +
as the angle which has a sine value of
 +
<math>{\sqrt{3}}/{2}\;</math>
 +
and then we have the reflectionally symmetric solution
 +
<math>x=\pi -\frac{\pi }{3}=\frac{2\pi }{3}</math>
 +
in the second quadrant.
 +
 +
Each of those solutions returns to itself after every revolution, so that we obtain the complete solution if we add multiples of
 +
<math>2\pi </math>
 +
 +
 +
 +
<math>x=\frac{\pi }{3}+2n\pi </math>
 +
and
 +
<math>x=\frac{2\pi }{3}+2n\pi </math>
 +
 +
 +
where
 +
<math>n</math>
 +
is an arbitrary integer.
 +
 +
NOTE: when we write that the complete solution is given by
 +
 +
 +
<math>x=\frac{\pi }{3}+2n\pi </math>
 +
and
 +
<math>x=\frac{2\pi }{3}+2n\pi </math>,
 +
 +
this means that for every integer
 +
<math>n</math>, we obtain a solution to the equation:
 +
 +
 +
<math>\begin{array}{*{35}l}
 +
n=0 & x=\frac{\pi }{3} & x=\frac{2\pi }{3} \\
 +
n=-1 & x=\frac{\pi }{3}+\left( -1 \right)\centerdot 2\pi & x=\frac{2\pi }{3}+\left( -1 \right)\centerdot 2\pi \\
 +
n=1 & x=\frac{\pi }{3}+1\centerdot 2\pi & x=\frac{2\pi }{3}+1\centerdot 2\pi \\
 +
n=-2 & x=\frac{\pi }{3}+\left( -2 \right)\centerdot 2\pi & x=\frac{2\pi }{3}+\left( -2 \right)\centerdot 2\pi \\
 +
n=2 & x=\frac{\pi }{3}+2\centerdot 2\pi & x=\frac{2\pi }{3}+2\centerdot 2\pi \\
 +
\end{array}</math>
 +
 +
and so on.

Revision as of 13:30, 30 September 2008

We draw a unit circle and mark on those angles on the circle which have a \displaystyle y -coordinate of \displaystyle {\sqrt{3}}/{2}\;, in order to see which solutions lie between \displaystyle 0 and \displaystyle 2\pi .


In the first quadrant, we recognize \displaystyle x={\pi }/{3}\; as the angle which has a sine value of \displaystyle {\sqrt{3}}/{2}\; and then we have the reflectionally symmetric solution \displaystyle x=\pi -\frac{\pi }{3}=\frac{2\pi }{3} in the second quadrant.

Each of those solutions returns to itself after every revolution, so that we obtain the complete solution if we add multiples of \displaystyle 2\pi


\displaystyle x=\frac{\pi }{3}+2n\pi and \displaystyle x=\frac{2\pi }{3}+2n\pi


where \displaystyle n is an arbitrary integer.

NOTE: when we write that the complete solution is given by


\displaystyle x=\frac{\pi }{3}+2n\pi and \displaystyle x=\frac{2\pi }{3}+2n\pi ,

this means that for every integer \displaystyle n, we obtain a solution to the equation:


\displaystyle \begin{array}{*{35}l} n=0 & x=\frac{\pi }{3} & x=\frac{2\pi }{3} \\ n=-1 & x=\frac{\pi }{3}+\left( -1 \right)\centerdot 2\pi & x=\frac{2\pi }{3}+\left( -1 \right)\centerdot 2\pi \\ n=1 & x=\frac{\pi }{3}+1\centerdot 2\pi & x=\frac{2\pi }{3}+1\centerdot 2\pi \\ n=-2 & x=\frac{\pi }{3}+\left( -2 \right)\centerdot 2\pi & x=\frac{2\pi }{3}+\left( -2 \right)\centerdot 2\pi \\ n=2 & x=\frac{\pi }{3}+2\centerdot 2\pi & x=\frac{2\pi }{3}+2\centerdot 2\pi \\ \end{array}

and so on.

Retrieved from "http://wiki.sommarmatte.se/wikis/2008/bridgecourse1-ImperialCollege/index.php/Solution_4.4:2a"